Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
We study both theoretically and numerically two-dimensional magnetohydrodynamic turbulence at infinite and zero magnetic Prandtl number 
$\mathit{Pm}$ (and the limits thereof), with an emphasis on solution regularity. For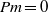 
$\mathit{Pm}= 0$, both 
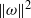$\Vert \omega \Vert ^{2} $ and 
$\Vert j\Vert ^{2} $, where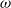 
$\omega $ and 
$j$ are, respectively, the vorticity and current, are uniformly bounded. Furthermore,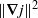 
$\Vert \boldsymbol{\nabla} j\Vert ^{2} $ is integrable over 
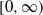$[0, \infty )$. The uniform boundedness of 
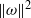$\Vert \omega \Vert ^{2} $ implies that in the presence of vanishingly small viscosity 
$\nu $ (i.e. in the limit 
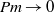$\mathit{Pm}\rightarrow 0$), the kinetic energy dissipation rate 
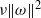$\nu \Vert \omega \Vert ^{2} $ vanishes for all times 
$t$, including 
$t= \infty $. Furthermore, for sufficiently small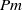 
$\mathit{Pm}$, this rate decreases linearly with 
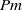$\mathit{Pm}$. This linear behaviour of 
$\nu \Vert \omega \Vert ^{2} $ is investigated and confirmed by high-resolution simulations with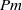 
$\mathit{Pm}$ in the range 
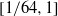$[1/ 64, 1] $. Several criteria for solution regularity are established and numerically tested. As 
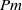$\mathit{Pm}$ is decreased from unity, the ratio 
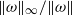$\Vert \omega \Vert _{\infty } / \Vert \omega \Vert $ is observed to increase relatively slowly. This, together with the integrability of 
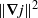$\Vert \boldsymbol{\nabla} j\Vert ^{2} $, suggests global regularity for 
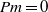$\mathit{Pm}= 0$. When 
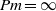$\mathit{Pm}= \infty $, global regularity is secured when either 
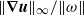$\Vert \boldsymbol{\nabla} \boldsymbol{u}\Vert _{\infty } / \Vert \omega \Vert $, where 
$\boldsymbol{u}$ is the fluid velocity, or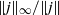 
$\Vert j\Vert _{\infty } / \Vert j\Vert $ is bounded. The former is plausible given the presence of viscous effects for this case. Numerical results over the range 
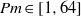$\mathit{Pm}\in [1, 64] $ show that 
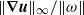$\Vert \boldsymbol{\nabla} \boldsymbol{u}\Vert _{\infty } / \Vert \omega \Vert $ varies slightly (with similar behaviour for 
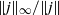$\Vert j\Vert _{\infty } / \Vert j\Vert $), thereby lending strong support for the possibility 
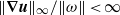$\Vert \boldsymbol{\nabla} \boldsymbol{u}\Vert _{\infty } / \Vert \omega \Vert \lt \infty $ in the limit 
$\mathit{Pm}\rightarrow \infty $. The peak of the magnetic energy dissipation rate 
$\mu \Vert j\Vert ^{2} $ is observed to decrease rapidly as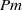 
$\mathit{Pm}$ is increased. This result suggests the possibility 
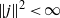$\Vert j\Vert ^{2} \lt \infty $ in the limit 
$\mathit{Pm}\rightarrow \infty $. We discuss further evidence for the boundedness of the ratios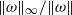 
$\Vert \omega \Vert _{\infty } / \Vert \omega \Vert $, 
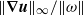$\Vert \boldsymbol{\nabla} \boldsymbol{u}\Vert _{\infty } / \Vert \omega \Vert $ and 
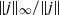$\Vert j\Vert _{\infty } / \Vert j\Vert $ in conjunction with observation on the density of filamentary structures in the vorticity, velocity gradient and current fields.
Open access
