Hostname: page-component-8448b6f56d-sxzjt Total loading time: 0 Render date: 2024-04-18T09:53:19.963Z Has data issue: false hasContentIssue false
  • English
  • Français

Weak approximation of stochastic differential delay equations for bounded measurable function

Part of: Stochastic analysis Functional-differential and differential-difference equations

Published online by Cambridge University Press:  01 September 2013

Hua Zhang
Hua Zhang*
Affiliation:
School of Statistics,Jiangxi University of Finance and Economics,Nanchang, Jiangxi 330013, PR China email zh860801@163.com School of Mathematics and Computational Science,Sun Yat-Sen University,Guangzhou, Guangdong 510275, PR China email zh860801@163.com

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we study the weak approximation problem of 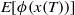$E[\phi (x(T))] $ by $E[\phi (y(T))] $, where 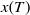$x(T)$ is the solution of a stochastic differential delay equation and 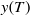$y(T)$ is defined by the Euler scheme. For 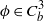$\phi \in { C}_{b}^{3} $, Buckwar, Kuske, Mohammed and Shardlow (‘Weak convergence of the Euler scheme for stochastic differential delay equations’, LMS J. Comput. Math. 11 (2008) 60–69) have shown that the Euler scheme has weak order of convergence 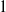$1$. Here we prove that the same results hold when 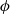$\phi $ is only assumed to be measurable and bounded under an additional non-degeneracy condition.

MSC classification

Secondary: 34K50: Stochastic functional-differential equations 60H07: Stochastic calculus of variations and the Malliavin calculus 60H35: Computational methods for stochastic equations
Type
Research Article
Information
LMS Journal of Computation and Mathematics , Volume 16 , October 2013 , pp. 319 - 343
Copyright
© The Author(s) 2013 

References

Buckwar, E., Kuske, R., Mohammed, S.-E. A. and Shardlow, T., ‘Weak convergence of the Euler scheme for stochastic differential delay equations’, LMS J. Comput. Math. 11 (2008) 60–69.CrossRefGoogle Scholar
Bell, D. R. and Mohammed, S. E. A., ‘The Malliavin calculus and stohastic delay equations’, J. Funct. Anal. 99 (1991) 75–99.CrossRefGoogle Scholar
Bally, V. and Talay, D., ‘The law of the Euler scheme for stochastic differential equations: I. Convergence rate of the distribution function’, Probab. Theory Related Fields 104 (1996) 43–60.CrossRefGoogle Scholar
Bally, V. and Talay, D., ‘The law of the Euler scheme for stochastic differential equations: II. Convergence rate of the rate of the density’, Monte Carlo Methods Appl. 2 (1996) 93–128.CrossRefGoogle Scholar
Bouleau, N. and Hirsch, F., Dirichlet forms and analysis on Wiener space, de Gruyter Studies in Mathematics (de Gruyter, Berlin, 1991).CrossRefGoogle Scholar
Clément, E., Kohatsu-Higa, A. and Lamberton, D., ‘A duality approach for the weak approximation of stochastic differential equations’, Ann. Appl. Probab. 16 (2006) 1124–1154.CrossRefGoogle Scholar
Huang, Z. and Yan, J., Introduction to infinite dimensional stochastic analysis, Mathematics and its Applications 502 (Kluwer Academic Publishers, Dordrecht, 2000). Translated and revised from the 1997 Chinese edition (Science Press, Beijing).CrossRefGoogle Scholar
Kloeden, P. E. and Platen, E., Numerical solutions of stochastic differential equations (Springer, New York, 1995).Google Scholar
Kohatsu-Higa, A., ‘Weak approximations, a Malliavin calculus approach’, Math. Comp. 70 (2001) 135–172.CrossRefGoogle Scholar
Kuosuoka, S. and Stroock, D., ‘Applications of the Malliavin calculus, Part I’, Stochastic analysis (Kata/Kyoto, 1982), North-Holland Math. Library 32 (North-Holland, Amsterdam, 1984) 271–306.Google Scholar
Malliavin, P., Stochastic analysis, Grundlehren der Mathematischen Wissenschaften 313 (Springer, Berlin, 1997).CrossRefGoogle Scholar
Mohammed, S. E. A., Stochastic functional differential equations, Research Notes in Mathematics 99 (Pitman, Boston, 1984).Google Scholar
Mohammed, S. E. A., ‘Stochastic differential systems with memory: theory, examples and applications’, Stochastic analysis, Progress in Probability 42 (eds Decreusefond, L., Gjerde, J., Øksendal, B. and Ustunel, A. S.; Birkhäuser, Basel, 1998) 1–77.Google Scholar
Nualart, D., ‘The Malliavin calculus and related topics’, Probability and its Applications, 2nd edn. (Springer, Berlin, 2006).Google Scholar
Nualart, D. and Pardoux, E., ‘Stochastic calculus with anticipating integrands’, Probab. Theory Related Fields 78 (1998) 535–581.CrossRefGoogle Scholar
He, K., Ren, J. and Zhang, H., ‘Localization of fractional Wiener functionals and applications’, Preprint, 2013, arXiv:1304.4316.Google Scholar