Hostname: page-component-8448b6f56d-jr42d Total loading time: 0 Render date: 2024-04-16T16:38:15.768Z Has data issue: false hasContentIssue false
  • English
  • Français

Invariants of degree 3 and torsion in the Chow group of a versal flag

Part of: Special varieties (Co)homology theory Forms and linear algebraic groups

Published online by Cambridge University Press:  16 April 2015

Alexander Merkurjev ,
Alexander Neshitov  and
Kirill Zainoulline
Alexander Merkurjev
Affiliation:
Department of Mathematics, University of California at Los Angeles, CA 90095, USA email merkurev@math.ucla.edu
Alexander Neshitov
Affiliation:
St. Petersburg Department of Steklov Mathematical Institute RAS, 27 Fontanka, 191023 St. Petersburg, Russia email neshitov@yandex.ru Department of Mathematics and Statistics, University of Ottawa, 585 King Edward, Ottawa, ON K1N 6N5, Canada
Kirill Zainoulline
Affiliation:
Department of Mathematics and Statistics, University of Ottawa, 585 King Edward, Ottawa, ON K1N 6N5, Canada email kirill@uottawa.ca
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We prove that the group of normalized cohomological invariants of degree 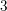$3$ modulo the subgroup of semidecomposable invariants of a semisimple split linear algebraic group 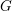$G$ is isomorphic to the torsion part of the Chow group of codimension-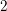$2$ cycles of the respective versal 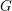$G$-flag. In particular, if 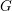$G$ is simple, we show that this factor group is isomorphic to the group of indecomposable invariants of 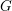$G$. As an application, we construct nontrivial cohomological invariants for indecomposable central simple algebras.

Keywords

cohomological invariantlinear algebraic grouptorsorGalois cohomologyChow group

MSC classification

Primary: 11E72: Galois cohomology of linear algebraic groups 14M17: Homogeneous spaces and generalizations 14F43: Other algebro-geometric (co)homologies (e.g., intersection, equivariant, Lawson, Deligne (co)homologies)
Type
Research Article
Information
Compositio Mathematica , Volume 151 , Issue 8 , August 2015 , pp. 1416 - 1432
Copyright
© The Authors 2015 

References

Barry, D., Decomposable and indecomposable algebras of degree 8 and exponent 2, Math. Z. 276 (2014), 1113–1132.CrossRefGoogle Scholar
Bermudez, H. and Ruozzi, A., Degree three cohomological invariants of groups that are neither simply-connected nor adjoint, Preprint (2013), arXiv:1305.2899v3.Google Scholar
Blinstein, S. and Merkurjev, A., Cohomological invariants of algebraic tori, Algebra Number Theory 7 (2013), 1643–1684.CrossRefGoogle Scholar
Colliot-Thélène, J.-L., Hoobler, R. and Kahn, B., The Bloch–Ogus–Gabber theorem, in Algebraic K-theory, Fields Institute Communications, vol. 16 (American Mathematical Society, Providence, RI, 1997), 31–94.Google Scholar
Edidin, D. and Graham, W., Equivariant intersection theory, Invent. Math. 131 (1998), 595–634.CrossRefGoogle Scholar
Fulton, W., Intersection theory, Ergebnisse der Mathematik und ihrer Grenzgebiete 3 [Series of Modern Surveys in Mathematics], vol. 2, second edition (Springer, Berlin, 1998).CrossRefGoogle Scholar
Garibaldi, S., Merkurjev, A. and Serre, J.-P., Cohomological invariants in Galois cohomology, University Lecture Series, vol. 28 (American Mathematical Society, Providence, RI, 2003).CrossRefGoogle Scholar
Garibaldi, S., Parimala, R. and Tignol, J.-P., Discriminant of symplectic involutions, Pure Appl. Math. Q. 5 (2009), 349–374.CrossRefGoogle Scholar
Garibaldi, S. and Zainoulline, K., The gamma-filtration and the Rost invariant, J. Reine Angew. Math. 696 (2014), 225–244.CrossRefGoogle Scholar
Gille, S. and Zainoulline, K., Equivariant pretheories and invariants of torsors, Transform. Groups 17 (2012), 471–498.CrossRefGoogle Scholar
Gros, M. and Suwa, N., La conjecture de Gersten pour les faisceaux de Hodge–Witt logarithmique, Duke Math. J. 57 (1988), 615–628.CrossRefGoogle Scholar
Kahn, B., Application of weight two motivic cohomology, Doc. Math. 1 (1996), 395–416.CrossRefGoogle Scholar
Karpenko, N., Codimension 2 cycles on Severi-Brauer varieties, K-Theory 13 (1998), 305–330.CrossRefGoogle Scholar
Knus, M.-A., Merkurjev, A., Rost, M. and Tignol, J.-P., The book of involutions, AMS Colloquium Publications, vol. 44 (American Mathematical Society, Providence, RI, 1998).CrossRefGoogle Scholar
Merkurjev, A., Degree three cohomological invariants of semisimple groups, J. Eur. Math. Soc., to appear, LAG Preprints no. 495.Google Scholar
Merkurjev, A., Weight two motivic cohomology of classifying spaces for semisimple groups, Preprint (2013), LAG Preprints no. 494.Google Scholar
Merkurjev, A., The group H 1(X, K 2) for projective homogeneous varieties, Algebra i Analiz 7 (1995), 136–164; translation in St. Petersburg Math. J. 7 (1996), 421–444.Google Scholar
Merkurjev, A., Equivariant K-theory, in Handbook of K-theory (Springer, Berlin, 2005), 925–954.CrossRefGoogle Scholar
Merkurjev, A. and Tignol, J.-P., The multipliers of similitudes and the Brauer group of homogeneous varieties, J. Reine Angew. Math. 461 (1995), 13–47.Google Scholar
Panin, I., On the algebraic K-theory of twisted flag varieties, K-Theory 8 (1994), 541–585.CrossRefGoogle Scholar
Peyre, E., Galois cohomology in degree three and homogeneous varieties, K-Theory 15 (1998), 99–145.CrossRefGoogle Scholar
Steinberg, R., On a theorem of Pittie, Topology 14 (1975), 173–177.CrossRefGoogle Scholar
Tits, J., Représentations linéaires irréductibles d’un groupe réductif sur un corps quelconque, J. Reine Angew. Math. 247 (1971), 196–220 (in French).Google Scholar
Zainoulline, K., Twisted gamma-filtration of a linear algebraic group, Compositio Math. 148 (2012), 1645–1654.CrossRefGoogle Scholar