Hostname: page-component-8448b6f56d-tj2md Total loading time: 0 Render date: 2024-04-19T15:23:33.469Z Has data issue: false hasContentIssue false
  • English
  • Français

Classification and rigidity of totally periodic pseudo-Anosov flows in graph manifolds

Published online by Cambridge University Press:  03 July 2014

THIERRY BARBOT  and
SÉRGIO R. FENLEY
THIERRY BARBOT
Affiliation:
Université d’Avignon et des pays de Vaucluse, LANLG, Faculté des Sciences, 33 rue Louis Pasteur, 84000 Avignon, France email thierry.barbot@univ-avignon.fr
SÉRGIO R. FENLEY
Affiliation:
Florida State University, Tallahassee, FL 32306-4510, USA Princeton University, Princeton, NJ 08544-1000, USA email fenley@math.princeton.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

In this article we analyze totally periodic pseudo-Anosov flows in graph 3-manifolds. This means that in each Seifert fibered piece of the torus decomposition, the free homotopy class of regular fibers has a finite power which is also a finite power of the free homotopy class of a closed orbit of the flow. We show that each such flow is topologically equivalent to one of the model pseudo-Anosov flows which we previously constructed in Barbot and Fenley (Pseudo-Anosov flows in toroidal manifolds. Geom. Topol. 17 (2013), 1877–1954). A model pseudo-Anosov flow is obtained by glueing standard neighborhoods of Birkhoff annuli and perhaps doing Dehn surgery on certain orbits. We also show that two model flows on the same graph manifold are isotopically equivalent (i.e. there is a isotopy of 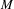$M$ mapping the oriented orbits of the first flow to the oriented orbits of the second flow) if and only if they have the same topological and dynamical data in the collection of standard neighborhoods of the Birkhoff annuli.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 35 , Issue 6 , September 2015 , pp. 1681 - 1722
Copyright
© Cambridge University Press, 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anosov, D. V.. Geodesic Flows on Closed Riemannian Manifolds with Negative Curvature (Proceedings of the Steklov Institute of Mathematics, 90). American Mathematical Society, Providence, RI, 1969.Google Scholar
Barbot, T.. Caractérisation des flots d’Anosov en dimension 3 par leurs feuilletages faibles. Ergod. Th. & Dynam. Sys. 15 (1995), 247–270.CrossRefGoogle Scholar
Barbot, T.. Flots d’Anosov sur les variétés graphées au sens de Waldhausen. Ann. Inst. Fourier (Grenoble) 46 (1996), 1451–1517.CrossRefGoogle Scholar
Barbot, T.. Mise en position optimale d’un tore par rapport à un flot d’Anosov. Comment. Math. Helv. 70 (1995), 113–160.CrossRefGoogle Scholar
Barbot, T. and Fenley, S.. Pseudo-Anosov flows in toroidal manifolds. Geom. Topol. 17 (2013), 1877–1954.CrossRefGoogle Scholar
Bonatti, C. and Langevin, R.. Un exemple de flot d’Anosov transitif transverse à un tore et non conjugué à une suspension. Ergod. Th. & Dynam. Sys. 14 (1994), 633–643.CrossRefGoogle Scholar
Brittenham, M., Naimi, R. and Roberts, R.. Graph manifolds and taut foliations. J. Differential Geom. 45 (1997), 446–470.CrossRefGoogle Scholar
Calegari, D.. The geometry of R -covered foliations. Geom. Topol. 4 (2000), 457–515.CrossRefGoogle Scholar
Calegari, D.. Foliations with one sided branching. Geom. Dedicata 96 (2003), 1–53.CrossRefGoogle Scholar
Calegari, D.. Promoting essential laminations. Invent. Math. 166 (2006), 583–643.CrossRefGoogle Scholar
Fenley, S.. Anosov flows in 3-manifolds. Ann. of Math. 139 (1994), 79–115.CrossRefGoogle Scholar
Fenley, S.. The structure of branching in Anosov flows of 3-manifolds. Comm. Math. Helv. 73 (1998), 259–297.CrossRefGoogle Scholar
Fenley, S.. Foliations with good geometry. J. Amer. Math. Soc. 12 (1999), 619–676.CrossRefGoogle Scholar
Fenley, S.. Foliations and the topology of 3-manifolds I: R -covered foliations and transverse pseudo-Anosov flows. Comm. Math. Helv. 77 (2002), 415–490.CrossRefGoogle Scholar
Fenley, S. and Mosher, L.. Quasigeodesic flows in hyperbolic 3-manifolds. Topology 40 (2001), 503–537.CrossRefGoogle Scholar
Franks, J. and Williams, R.. Anomalous Anosov flows. Global Theory of Dynamic Systems (Lecture Notes in Mathematics, 819). Springer, Berlin, 1980.Google Scholar
Fried, D.. Transitive Anosov flows and pseudo-Anosov maps. Topology 22 (1983), 299–303.CrossRefGoogle Scholar
Ghys, E.. Flots d’Anosov sur les 3-variétés fibrées en cercles. Ergod. Th. & Dynam. Sys. 4 (1984), 67–80.CrossRefGoogle Scholar
Goodman, S.. Dehn Surgery on Anosov Flows (Lecture Notes in Mathematics, 1007). Springer, Berlin, 1983, pp. 300–307.Google Scholar
Haefliger, A.. Groupöides d’holonomie et classifiants. Asterisque 116 (1984), 70–97.Google Scholar
Handel, M. and Thurston, W.. Anosov flows on new three manifolds. Invent. Math. 59 (1980), 95–103.CrossRefGoogle Scholar
Hempel, J.. 3-manifolds (Annals of Mathematical Studies, 86). Princeton University Press, Princeton, NJ, 1976.Google Scholar
Jaco, W.. Lectures on Three-manifold Topology (CBMS Regional Conference Series in Mathematics, 43). American Mathematical Society, Providence, RI, 1980.CrossRefGoogle Scholar
Jaco, W. and Shalen, P.. Seifert Fibered Spaces in 3-manifolds (Memoirs of the American Mathematical Society, 220). American Mathematical Society, Providence, RI, 1979.CrossRefGoogle Scholar
Johannson, K.. Homotopy Equivalences of 3-manifolds With Boundaries (Lecture Notes in Mathematics, 761). Springer, Berlin, 1979.CrossRefGoogle Scholar
Matsumoto, S. and Tsuboi, T.. Transverse intersection of foliations in three-manifolds. Mon. Enseign. Math. 38 (2001), 503–525.Google Scholar
Mosher, L.. Dynamical systems and the homology norm of a 3-manifold. I. Efficient intersection of surfaces and flows. Duke Math. J. 65 (1992), 449–500.CrossRefGoogle Scholar
Mosher, L.. Dynamical systems and the homology norm of a 3-manifold II. Invent. Math. 107 (1992), 243–281.CrossRefGoogle Scholar
Mosher, L.. Laminations and flows transverse to finite depth foliations, manuscript available in the web from http://newark.rutgers.edu:80/mosher/, Part I, Branched surfaces and dynamics; Part II, in preparation.Google Scholar
Seifert, H.. Topologie dreidimensionaler gefäserter raume. Acta Math. 60 147–238.CrossRefGoogle Scholar
Thurston, W.. The Geometry and Topology of 3-manifolds (Princeton University Lecture Notes). Princeton University Press, Princeton, NJ, 1982.Google Scholar
Thurston, W.. On the geometry and dynamics of diffeomorphisms of surfaces. Bull. Amer. Math. Soc. 19 (1988), 417–431.CrossRefGoogle Scholar
Thurston, W..  Hyperbolic structures on 3-manifolds II, Surface groups and 3-manifolds that fiber over the circle. Preprint.Google Scholar
Waller, R.. Surfaces which are flow graphs. Preprint, 2014.Google Scholar
Waldhausen, F.. Eine Klasse von 3-dimensionalen Mannigfaltigkeiten, I. Invent. Math. 3 (1967), 308–333.CrossRefGoogle Scholar
Waldhausen, F.. Eine Klasse von 3-dimensionalen Mannigfaltigkeiten, II. Invent. Math. 4 (1967), 87–117.CrossRefGoogle Scholar
Waldhausen, F.. On irreducible 3-manifolds which are sufficiently large. Ann. of Math. 87 (1968), 56–88.CrossRefGoogle Scholar