Article contents
Forcing the failure of CH by adding a real
Published online by Cambridge University Press: 12 March 2014
Extract
We prove several independence results relevant to an old question in the folklore of set theory. These results complement those in [Sh, Chapter XIII, §4]. The question is the following. Suppose V ⊨ “ZFC + CH” and r is a real not in V. Must V[r] ⊨ CH? To avoid trivialities assume 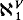 =
= 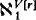 .
.
We answer this question negatively. Specifically we find pairs of models (W, V) such that W ⊨ ZFC + CH, V = W[r], r a real,  =
= 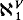 and V ⊨ ¬CH. Actually we find a spectrum of such pairs using ZFC up to “ZFC + there exist measurable cardinals”. Basically the nicer the pair is as a solution, the more we need to assume in order to construct it.
and V ⊨ ¬CH. Actually we find a spectrum of such pairs using ZFC up to “ZFC + there exist measurable cardinals”. Basically the nicer the pair is as a solution, the more we need to assume in order to construct it.
The relevant results in [Sh, Chapter XIII] state that if a pair (of inner models) (W, V) satisfies (1) and (2) then there is an inaccessible cardinal in L; if in addition V ⊨ 2ℵ0 > ℵ2 then 0# exists; and finally if (W, V) satisfies (1), (2) and (3) with V ⊨ 2ℵ0 > ℵω, then there is an inner model with a measurable cardinal.
Definition 1. For a pair (W, V) we shall consider the following conditions:
(1) V = W[r], r a real, 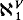 =
=  , W ⊨ ZFC + CH but CH fails in V.
, W ⊨ ZFC + CH but CH fails in V.
(2) W ⊨ GCH.
(3) W and V have the same cardinals.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1984
References
REFERENCES
- 9
- Cited by




