Article contents
Classifying model-theoretic properties
Published online by Cambridge University Press: 12 March 2014
Abstract
In 2004 Csima, Hirschfeldt, Knight, and Soare [1] showed that a set A ≤T 0′ is nonlow2 if and only if A is prime bounding, i.e., for every complete atomic decidable theory T, there is a prime model  computable in A. The authors presented nine seemingly unrelated predicates of a set A, and showed that they are equivalent for
computable in A. The authors presented nine seemingly unrelated predicates of a set A, and showed that they are equivalent for 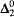 sets. Some of these predicates, such as prime bounding, and others involving equivalence structures and abelian p-groups come from model theory, while others involving meeting dense sets in trees and escaping a given function come from pure computability theory.
sets. Some of these predicates, such as prime bounding, and others involving equivalence structures and abelian p-groups come from model theory, while others involving meeting dense sets in trees and escaping a given function come from pure computability theory.
As predicates of A, the original nine properties are equivalent for 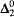 sets; however, they are not equivalent in general. This article examines the (degree-theoretic) relationship between the nine properties. We show that the nine properties fall into three classes, each of which consists of several equivalent properties. We also investigate the relationship between the three classes, by determining whether or not any of the predicates in one class implies a predicate in another class.
sets; however, they are not equivalent in general. This article examines the (degree-theoretic) relationship between the nine properties. We show that the nine properties fall into three classes, each of which consists of several equivalent properties. We also investigate the relationship between the three classes, by determining whether or not any of the predicates in one class implies a predicate in another class.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 2008
References
REFERENCES
- 4
- Cited by




