Article contents
Compactness properties of critical nonlinearities and nonlinear Schrödinger equations
Published online by Cambridge University Press: 05 April 2013
Abstract
We prove the compactness of critical Sobolev embeddings with applications to nonlinear singular Schrödinger equations and provide a unified treatment in dimensions N > 2 and N = 2, based on a somewhat unexpectedly broad array of parallel properties between spaces 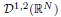 and H10 of the unit disc. These properties include Leray inequality for N = 2 as a counterpart of Hardy inequality for N > 2, pointwise estimates by ground states r(2−N)/2 and
and H10 of the unit disc. These properties include Leray inequality for N = 2 as a counterpart of Hardy inequality for N > 2, pointwise estimates by ground states r(2−N)/2 and 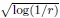 of the respective Hardy-type inequalities, as well as compactness of the limiting Sobolev embeddings once the Sobolev norm is appended by a potential term whose growth at singularities exceeds that of the corresponding Hardy-type potential.
of the respective Hardy-type inequalities, as well as compactness of the limiting Sobolev embeddings once the Sobolev norm is appended by a potential term whose growth at singularities exceeds that of the corresponding Hardy-type potential.
Keywords
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 2013
References
- 6
- Cited by




