Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Fitzgibbon, W.E.
1982.
Nonlinear Phenomena in Mathematical Sciences.
p.
389.
Krein, S. G.
and
Khazan, M. I.
1985.
Differential equations in a Banach space.
Journal of Soviet Mathematics,
Vol. 30,
Issue. 3,
p.
2154.
Solà-Morales, J.
1986.
Global instability and essential spectrum in semilinear evolution equations.
Mathematische Annalen,
Vol. 274,
Issue. 1,
p.
125.
Grabowski, Piotr
1986.
System Modelling and Optimization.
Vol. 84,
Issue. ,
p.
282.
Garay, B. M.
1988.
Parallelisability in Banach spaces: a parallelisable dynamical system with uniformly bounded trajectories.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 108,
Issue. 3-4,
p.
371.
Slemrod, M.
1988.
The 'relaxed invariance principle' and its applications.
p.
373.
Haraux, Alain
1988.
Attractors of asymptotically compact processes and applications to nonlinear partial differential equations.
Communications in Partial Differential Equations,
Vol. 13,
Issue. 11,
p.
1383.
Slemrod, M.
1989.
Weak asymptotic decay via a “relaxed invariance principle” for a wave equation with nonlinear, non-monotone damping.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 113,
Issue. 1-2,
p.
87.
Haraux, Alain
2001.
Remarks on weak stabilization of semilinear wave equations.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 6,
Issue. ,
p.
553.
Raugel, G.
2002.
Vol. 2,
Issue. ,
p.
885.
Esquivel-Avila, Jorge A
2003.
The dynamics of a nonlinear wave equation.
Journal of Mathematical Analysis and Applications,
Vol. 279,
Issue. 1,
p.
135.
Chill, R.
and
Jendoubi, M.A.
2003.
Convergence to steady states in asymptotically autonomous semilinear evolution equations.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 53,
Issue. 7-8,
p.
1017.
Chill, Ralph
and
Fašangová, Eva
2005.
Convergence to steady states of solutions of semilinear evolutionary integral equations.
Calculus of Variations and Partial Differential Equations,
Vol. 22,
Issue. 3,
p.
321.
Gazzola, Filippo
and
Squassina, Marco
2006.
Global solutions and finite time blow up for damped semilinear wave equations.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 23,
Issue. 2,
p.
185.
Babin, A.V.
2006.
Vol. 1,
Issue. ,
p.
983.
WU, HAO
GRASSELLI, MAURIZIO
and
ZHENG, SONGMU
2007.
CONVERGENCE TO EQUILIBRIUM FOR A PARABOLIC–HYPERBOLIC PHASE-FIELD SYSTEM WITH NEUMANN BOUNDARY CONDITIONS.
Mathematical Models and Methods in Applied Sciences,
Vol. 17,
Issue. 01,
p.
125.
Wu, Hao
Grasselli, Maurizio
and
Zheng, Songmu
2007.
Convergence to equilibrium for a parabolic–hyperbolic phase-field system with dynamical boundary condition.
Journal of Mathematical Analysis and Applications,
Vol. 329,
Issue. 2,
p.
948.
Carvalho, A.
and
Cholewa, J.
2008.
Local well posedness, asymptotic behavior and asymptotic bootstrapping for a class of semilinear evolution equations of the second order in time.
Transactions of the American Mathematical Society,
Vol. 361,
Issue. 5,
p.
2567.
Ducrot, A.
Liu, Z.
and
Magal, P.
2008.
Essential growth rate for bounded linear perturbation of non-densely defined Cauchy problems.
Journal of Mathematical Analysis and Applications,
Vol. 341,
Issue. 1,
p.
501.
CHILL, RALPH
HARAUX, ALAIN
and
JENDOUBI, MOHAMED ALI
2009.
APPLICATIONS OF THE ŁOJASIEWICZ–SIMON, GRADIENT INEQUALITY TO GRADIENT-LIKE EVOLUTION EQUATIONS.
Analysis and Applications,
Vol. 07,
Issue. 04,
p.
351.
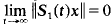 uniformly in bounded sets of x in X, and (2) S2(t) is compact for t sufficiently large. Then, if the orbit {S(t)x: t ≧0} of x ∈ X is bounded in X, it is precompact in X. Applications are made to an age dependent population model, a non-linear functional differential equation on an infinite interval, and a non-linear Volterra integrodifferential equation.
uniformly in bounded sets of x in X, and (2) S2(t) is compact for t sufficiently large. Then, if the orbit {S(t)x: t ≧0} of x ∈ X is bounded in X, it is precompact in X. Applications are made to an age dependent population model, a non-linear functional differential equation on an infinite interval, and a non-linear Volterra integrodifferential equation.



