Article contents
Hecke groups and continued fractions
Published online by Cambridge University Press: 17 April 2009
Abstract
The Hecke groups
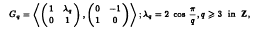
are Fuchsian groups of the first kind. In an interesting analogy to the use of ordinary continued fractions to study the geodesics of the modular surface, the λ-continued fractions (λF) introduced by the first author can be used to study those on the surfaces determined by the Gq. In this paper we focus on periodic continued fractions, corresponding to closed geodesics, and prove that the period of the λF for periodic 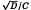 has nearly the form of the classical case. From this, we give: (1) a necessary and sufficient condition for
has nearly the form of the classical case. From this, we give: (1) a necessary and sufficient condition for 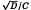 to be periodic; (2) examples of elements of ℚ(λq) which also have such periodic expansions; (3) a discussion of solutions to Pell's equation in quadratic extensions of the ℚ(λq); and (4) Legendre's constant of diophantine approximation for the Gq, that is, γq such that
to be periodic; (2) examples of elements of ℚ(λq) which also have such periodic expansions; (3) a discussion of solutions to Pell's equation in quadratic extensions of the ℚ(λq); and (4) Legendre's constant of diophantine approximation for the Gq, that is, γq such that 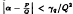 < γq/Q2 implies that P/Q of “reduced finite λF form” is a convergent of real α ∉ Gq(∞).
< γq/Q2 implies that P/Q of “reduced finite λF form” is a convergent of real α ∉ Gq(∞).
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 46 , Issue 3 , December 1992 , pp. 459 - 474
- Copyright
- Copyright © Australian Mathematical Society 1992
References
- 8
- Cited by




