Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Aleman, Alexandru
and
Constantin, Olivia
2009.
Spectra of integration operators on weighted bergman spaces.
Journal d'Analyse Mathématique,
Vol. 109,
Issue. 1,
p.
199.
Aleman, Alexandru
and
Persson, Anna-Maria
2010.
Resolvent estimates and decomposable extensions of generalized Cesàro operators.
Journal of Functional Analysis,
Vol. 258,
Issue. 1,
p.
67.
Pau, Jordi
and
Peláez, José Ángel
2010.
Embedding theorems and integration operators on Bergman spaces with rapidly decreasing weights.
Journal of Functional Analysis,
Vol. 259,
Issue. 10,
p.
2727.
Laitila, Jussi
Miihkinen, Santeri
and
Nieminen, Pekka J.
2011.
Essential norms and weak compactness of integration operators.
Archiv der Mathematik,
Vol. 97,
Issue. 1,
p.
39.
Sharma, S. D.
and
Sharmat, Anshu
2011.
Generalized integration operators from Bloch type spaces to weighted BMOA Spaces.
Demonstratio Mathematica,
Vol. 44,
Issue. 2,
Sharma, Anshu
and
Sharma, Ajay K.
2011.
Carleson measures and a class of generalized integration operators on the Bergman space.
Rocky Mountain Journal of Mathematics,
Vol. 41,
Issue. 5,
HU, ZHANGJIAN
2012.
ESSENTIAL NORM OF EXTENDED CESÀRO OPERATORS FROM ONE BERGMAN SPACE TO ANOTHER.
Bulletin of the Australian Mathematical Society,
Vol. 85,
Issue. 2,
p.
307.
Liu, Junming
Lou, Zengjian
and
Xiong, Chengji
2012.
Essential norms of integral operators on spaces of analytic functions.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 75,
Issue. 13,
p.
5145.
Constantin, Olivia
and
Persson, Anna-Maria
2015.
The spectrum of Volterra-type integration operators on generalized Fock spaces.
Bulletin of the London Mathematical Society,
p.
bdv069.
Liu, Chao
2020.
Multipliers for Dirichlet type spaces by randomization.
Banach Journal of Mathematical Analysis,
Vol. 14,
Issue. 3,
p.
935.
Miihkinen, Santeri
Pau, Jordi
Perälä, Antti
and
Wang, Maofa
2020.
Volterra type integration operators from Bergman spaces to Hardy spaces.
Journal of Functional Analysis,
Vol. 279,
Issue. 4,
p.
108564.
KIZGUT, Ersin
2021.
Volterra operators between limits of Bergman-type weighted spaces of analytic functions.
Hacettepe Journal of Mathematics and Statistics,
Vol. 50,
Issue. 4,
p.
949.
Duan, Yongjiang
Wang, Siyu
and
Wang, Zipeng
2023.
Volterra-Type Integration Operators Between Weighted Bergman Spaces and Hardy Spaces.
Computational Methods and Function Theory,
Vol. 23,
Issue. 4,
p.
589.
Pang, Changbao
Perälä, Antti
Wang, Maofa
and
Guo, Xin
2023.
Maximal estimate and integral operators in Bergman spaces with doubling measure.
Proceedings of the American Mathematical Society,
Vol. 151,
Issue. 7,
p.
2881.
Jreis, Joelle
and
Lefèvre, Pascal
2024.
Some Operator Ideal Properties of Volterra Operators on Bergman and Bloch Spaces.
Integral Equations and Operator Theory,
Vol. 96,
Issue. 1,
 into another is established, and an asymptotic formula for the essential norm of Jg, the distance from compact operators in the operator norm, is given. As an immediate consequence it is obtained that if p < 2 + α and α > −1, then any primitive of
into another is established, and an asymptotic formula for the essential norm of Jg, the distance from compact operators in the operator norm, is given. As an immediate consequence it is obtained that if p < 2 + α and α > −1, then any primitive of 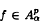 belongs to
belongs to  where q = ((2 + α) p)/(2 + α − p). For α = −1 this is a sharp result by Hardy and Littlewood on primitives of functions in Hardy space
where q = ((2 + α) p)/(2 + α − p). For α = −1 this is a sharp result by Hardy and Littlewood on primitives of functions in Hardy space 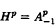 , 0 < p < 1.
, 0 < p < 1.



