Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bradley, Richard C.
1986.
Dependence in Probability and Statistics.
p.
165.
Philipp, Walter
1986.
Dependence in Probability and Statistics.
p.
225.
Bradley, Richard C.
and
Peligrad, Magda
1986.
Invariance principles under a two-part mixing assumption.
Stochastic Processes and their Applications,
Vol. 22,
Issue. 2,
p.
271.
Burton, Robert
and
Denker, Manfred
1987.
On the central limit theorem for dynamical systems.
Transactions of the American Mathematical Society,
Vol. 302,
Issue. 2,
p.
715.
Rudolph, Daniel J.
1988.
Asymptotically Brownian skew products give non-loosely BernoulliK-automorphisms.
Inventiones Mathematicae,
Vol. 91,
Issue. 1,
p.
105.
Taylor, Thomas J. S.
1989.
Computation and Control.
p.
333.
Katsuda, Atsushi
and
Sunada, Toshikazu
1989.
Dynamical 𝐿-functions and homology of closed orbits.
Bulletin of the American Mathematical Society,
Vol. 20,
Issue. 1,
p.
73.
Coelho, Zaqueu
and
Parry, William
1990.
Central limit asymptotics for shifts of finite type.
Israel Journal of Mathematics,
Vol. 69,
Issue. 2,
p.
235.
Katsuda, Atsushi
and
Sunada, Toshikazu
1990.
Closed orbits in homology classes.
Publications mathématiques de l'IHÉS,
Vol. 71,
Issue. 1,
p.
5.
Beck, Christian
1990.
Brownian motion from deterministic dynamics.
Physica A: Statistical Mechanics and its Applications,
Vol. 169,
Issue. 2,
p.
324.
Peligrad, Magda
1992.
On the central limit theorem for weakly dependent sequences with a decomposed strong mixing coefficient.
Stochastic Processes and their Applications,
Vol. 42,
Issue. 2,
p.
181.
Boyle, Mike
1993.
Combinatorial and Graph-Theoretical Problems in Linear Algebra.
Vol. 50,
Issue. ,
p.
1.
Taylor, Thomas j.
1993.
On stochastic and chaotic motion.
Stochastics and Stochastic Reports,
Vol. 43,
Issue. 3-4,
p.
179.
Pollicott, Mark
1994.
Derivatives of topological entropy for Anosov and geodesic flows.
Journal of Differential Geometry,
Vol. 39,
Issue. 3,
Kifer, Yuri
1995.
Limit theorems in averaging for dynamical systems.
Ergodic Theory and Dynamical Systems,
Vol. 15,
Issue. 6,
p.
1143.
Golse, François
1995.
Asymptotic Modelling in Fluid Mechanics.
Vol. 442,
Issue. ,
p.
179.
Beck, Christian
1996.
Dynamical systems of Langevin type.
Physica A: Statistical Mechanics and its Applications,
Vol. 233,
Issue. 1-2,
p.
419.
Waddington, Simon
1996.
Large deviation asymptotics for Anosov flows.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 13,
Issue. 4,
p.
445.
Bardos, Claude
Golse, François
and
Colonna, Jean-François
1997.
Diffusion approximation and hyperbolic automorphisms of the torus.
Physica D: Nonlinear Phenomena,
Vol. 104,
Issue. 1,
p.
32.
Bradley, Richard C.
1997.
Every “lower psi-mixing” Markov chain is “interlaced rho-mixing”.
Stochastic Processes and their Applications,
Vol. 72,
Issue. 2,
p.
221.
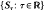 denote a flow built under a Hölder-continuous function l over the base (Σ, μ) where Σ is a topological Markov chain and μ some (ψ-mining) Gibbs measure. For a certain class of functions f with finite 2 + δ-moments it is shown that there exists a Brownian motion B(t) with respect to μ and σ2 > 0 such that μ-a.e.
denote a flow built under a Hölder-continuous function l over the base (Σ, μ) where Σ is a topological Markov chain and μ some (ψ-mining) Gibbs measure. For a certain class of functions f with finite 2 + δ-moments it is shown that there exists a Brownian motion B(t) with respect to μ and σ2 > 0 such that μ-a.e.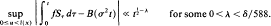
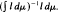 . From this, the central limit theorem, the weak invariance principle, the law of the iterated logarithm and related probabilistic results follow immediately. In particular, the result of Ratner ([6]) is extended.
. From this, the central limit theorem, the weak invariance principle, the law of the iterated logarithm and related probabilistic results follow immediately. In particular, the result of Ratner ([6]) is extended.



