No CrossRef data available.
Article contents
SETS WITH EVEN PARTITION FUNCTIONS AND CYCLOTOMIC NUMBERS
Published online by Cambridge University Press: 14 March 2016
Abstract
Let 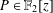 $P\in \mathbb{F}_{2}[z]$ be such that
$P\in \mathbb{F}_{2}[z]$ be such that 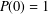 $P(0)=1$ and degree
$P(0)=1$ and degree  $(P)\geq 1$. Nicolas et al. [‘On the parity of additive representation functions’, J. Number Theory73 (1998), 292–317] proved that there exists a unique subset
$(P)\geq 1$. Nicolas et al. [‘On the parity of additive representation functions’, J. Number Theory73 (1998), 292–317] proved that there exists a unique subset 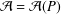 ${\mathcal{A}}={\mathcal{A}}(P)$ of
${\mathcal{A}}={\mathcal{A}}(P)$ of 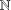 $\mathbb{N}$ such that
$\mathbb{N}$ such that 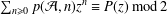 $\sum _{n\geq 0}p({\mathcal{A}},n)z^{n}\equiv P(z)~\text{mod}\,2$, where
$\sum _{n\geq 0}p({\mathcal{A}},n)z^{n}\equiv P(z)~\text{mod}\,2$, where 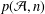 $p({\mathcal{A}},n)$ is the number of partitions of
$p({\mathcal{A}},n)$ is the number of partitions of  $n$ with parts in
$n$ with parts in 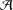 ${\mathcal{A}}$. Let
${\mathcal{A}}$. Let 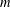 $m$ be an odd positive integer and let
$m$ be an odd positive integer and let 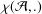 ${\it\chi}({\mathcal{A}},.)$ be the characteristic function of the set
${\it\chi}({\mathcal{A}},.)$ be the characteristic function of the set  ${\mathcal{A}}$. Finding the elements of the set
${\mathcal{A}}$. Finding the elements of the set 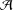 ${\mathcal{A}}$ of the form
${\mathcal{A}}$ of the form 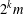 $2^{k}m$,
$2^{k}m$, 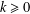 $k\geq 0$, is closely related to the
$k\geq 0$, is closely related to the 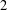 $2$-adic integer
$2$-adic integer  $S({\mathcal{A}},m)={\it\chi}({\mathcal{A}},m)+2{\it\chi}({\mathcal{A}},2m)+4{\it\chi}({\mathcal{A}},4m)+\cdots =\sum _{k=0}^{\infty }2^{k}{\it\chi}({\mathcal{A}},2^{k}m)$, which has been shown to be an algebraic number. Let
$S({\mathcal{A}},m)={\it\chi}({\mathcal{A}},m)+2{\it\chi}({\mathcal{A}},2m)+4{\it\chi}({\mathcal{A}},4m)+\cdots =\sum _{k=0}^{\infty }2^{k}{\it\chi}({\mathcal{A}},2^{k}m)$, which has been shown to be an algebraic number. Let 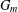 $G_{m}$ be the minimal polynomial of
$G_{m}$ be the minimal polynomial of  $S({\mathcal{A}},m)$. In precedent works there were treated the case
$S({\mathcal{A}},m)$. In precedent works there were treated the case 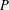 $P$ irreducible of odd prime order
$P$ irreducible of odd prime order 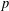 $p$. In this setting, taking
$p$. In this setting, taking 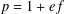 $p=1+ef$, where
$p=1+ef$, where 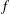 $f$ is the order of
$f$ is the order of 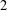 $2$ modulo
$2$ modulo 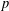 $p$, explicit determinations of the coefficients of
$p$, explicit determinations of the coefficients of 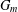 $G_{m}$ have been made for
$G_{m}$ have been made for 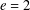 $e=2$ and 3. In this paper, we treat the case
$e=2$ and 3. In this paper, we treat the case 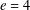 $e=4$ and use the cyclotomic numbers to make explicit
$e=4$ and use the cyclotomic numbers to make explicit 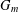 $G_{m}$.
$G_{m}$.
Keywords
MSC classification
- Type
- Research Article
- Information
- Copyright
- © 2016 Australian Mathematical Publishing Association Inc.




