Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bismut, Jean-Michel
2016.
Toeplitz Operators, Analytic Torsion, and the Hypoelliptic Laplacian.
Letters in Mathematical Physics,
Vol. 106,
Issue. 12,
p.
1639.
So, Bing Kwan
and
Su, GuangXiang
2017.
Regularity of the analytic torsion form on families of normal coverings.
Pacific Journal of Mathematics,
Vol. 291,
Issue. 1,
p.
149.
Finski, Siarhei
2018.
On the full asymptotics of analytic torsion.
Journal of Functional Analysis,
Vol. 275,
Issue. 12,
p.
3457.
Shen, Shu
2018.
Analytic torsion, dynamical zeta functions, and the Fried conjecture.
Analysis & PDE,
Vol. 11,
Issue. 1,
p.
1.
Albin, Pierre
Rochon, Frédéric
and
Sher, David
2018.
Analytic torsion and R-torsion of Witt representations on manifolds with cusps.
Duke Mathematical Journal,
Vol. 167,
Issue. 10,
Liu, Bingxiao
2018.
Hypoelliptic Laplacian and twisted trace formula.
Comptes Rendus. Mathématique,
Vol. 357,
Issue. 1,
p.
74.
Müller, Werner
and
Rochon, Frédéric
2021.
Exponential growth of torsion in the cohomology of arithmetic hyperbolic manifolds.
Mathematische Zeitschrift,
Vol. 298,
Issue. 1-2,
p.
79.
Shen, Shu
2021.
Analytic Torsion, Dynamical Zeta Function, and the Fried Conjecture for Admissible Twists.
Communications in Mathematical Physics,
Vol. 387,
Issue. 2,
p.
1215.
Liu, Bingxiao
2021.
Asymptotic equivariant real analytic torsions for compact locally symmetric spaces.
Journal of Functional Analysis,
Vol. 281,
Issue. 7,
p.
109117.
Zhang, Yeping
2022.
The direct image of a flat fibration with complex fibers.
Bulletin des Sciences Mathématiques,
Vol. 176,
Issue. ,
p.
103115.
Albin, Pierre
Rochon, Frédéric
and
Sher, David
2022.
A Cheeger–Müller theorem for manifolds with
wedge singularities.
Analysis & PDE,
Vol. 15,
Issue. 3,
p.
567.
Ma, Xiaonan
and
Zhang, Weiping
2023.
Superconnection and family Bergman kernels.
Mathematische Annalen,
Vol. 386,
Issue. 3-4,
p.
2207.
Liu, Bingxiao
2023.
Hypoelliptic Laplacian and twisted trace formula.
Annales de l'Institut Fourier,
Vol. 73,
Issue. 5,
p.
1909.
Puchol, Martin
2023.
The asymptotics of the holomorphic analytic torsion forms.
Journal of the London Mathematical Society,
Vol. 108,
Issue. 1,
p.
80.
Ma, Qiaochu
2024.
Toeplitz operators and the full asymptotic torsion forms.
Journal of Functional Analysis,
Vol. 286,
Issue. 3,
p.
110210.
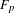 $F_{p}$
. For
$F_{p}$
. For
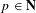 $p\in \mathbf{N}$
, the flat vector bundle
$p\in \mathbf{N}$
, the flat vector bundle
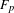 $F_{p}$
is the direct image of
$F_{p}$
is the direct image of
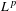 $L^{p}$
, where
$L^{p}$
, where
 $L$
is a holomorphic positive line bundle on the fibres of a flat fibration by compact Kähler manifolds. The leading term of the analytic torsion forms is the integral along the fibre of a locally defined differential form.
$L$
is a holomorphic positive line bundle on the fibres of a flat fibration by compact Kähler manifolds. The leading term of the analytic torsion forms is the integral along the fibre of a locally defined differential form.



